NCERT Solutions Ch-6 The Triangle and its Properties
Chapter 6 of the NCERT Class 7 Mathematics textbook, titled “The Triangle and its Properties,” delves into the fundamental aspects of triangles, including their definitions, types, and essential properties. It introduces various types of triangles based on their sides—scalene, isosceles, and equilateral—and based on their angles—acute, right, and obtuse. The chapter emphasizes important properties, such as the sum of the interior angles of a triangle always being 180 degrees, and discusses the concept of congruence among triangles. Through engaging examples and practical applications, students learn to recognize and classify triangles, explore the relationships between their sides and angles, and apply these principles to solve geometric problems. Overall, the chapter lays a solid foundation for understanding more complex geometric concepts in the future.
NCERT Solutions of Chapter 6 The Triangle and its Properties exercise 6.1, 6.2 , 6.3, 6.4 and 6.5
We try to teach you all Questions in easy way. We solve all chapter wise sums of maths textbook. In every chapter include NCERT solutions. For solutions of Exercise 6.1, 6.2, 6.3, 6.4 and 6.5 click on Tabs :
NCERT Solutions of class 7th maths Chapter 6 The Triangle and its Properties Exercise 6.1
Q1. In Δ PQR, D is the mid-point of ![]() .
.
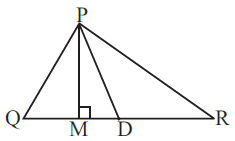
(i) ![]() is ___.
is ___.
Solution:-
Altitude
An altitude has one end point at a vertex of the triangle and other on the line containing the opposite side.
(ii) PD is ___.
Solution:-
Median
A median connects a vertex of a triangle to the mid-point of the opposite side.
(iii) Is QM = MR?
Solution:-
No, QM ≠ MR because, D is the mid-point of QR.
2. Draw rough sketches for the following:
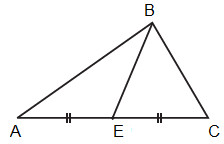
(a) In ΔABC, BE is a median.
Solution:-
A median connects a vertex of a triangle to the mid-point of the opposite side.
(b) In ΔPQR, PQ and PR are altitudes of the triangle.
Solution:-
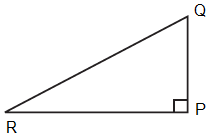
An altitude has one end point at a vertex of the triangle and other on the line containing the opposite side.
(c) In ΔXYZ, YL is an altitude in the exterior of the triangle.
Solution:-
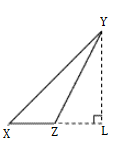
In the figure we may observe that for ΔXYZ, YL is an altitude drawn exteriorly to side XZ which is extended up to point L.
3. Verify by drawing a diagram if the median and altitude of an isosceles triangle can be same.
Solution:-
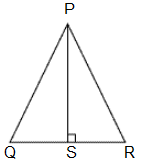
Draw a Line segment PS ⊥ BC. It is an altitude for this triangle. Here we observe that length of QS and SR is also same. So PS is also a median of this triangle.
NCERT Solutions of class 7th maths Chapter 6 The Triangle and its Properties Exercise 6.2
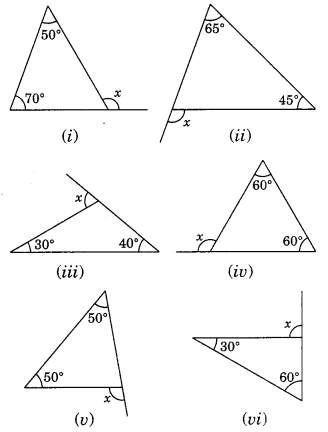
Q1. Find the value of the unknown exterior angle x in the following diagram:
Solution:
(i) ∠x = 50° + 70° = 120° (Exterior angle is equal to sum of its interior opposite angles)
(ii) ∠x = 65°+ 45° = 110° (Exterior angle is equal to sum of its interior opposite angles)
(iii) ∠x = 30° + 40° = 70° (Exterior angle is equal to sum of its interior opposite angles)
(iv) ∠x = 60° + 60° = 120° (Exterior angle is equal to sum of its interior opposite angles)
(v) ∠x = 50° + 50° =100° (Exterior angle is equal to sum of its interior opposite angles)
(vi) ∠x = 30° + 60° = 90° (Exterior angle is equal to sum of its interior opposite angle)
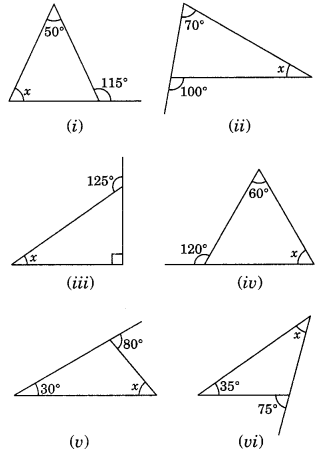
2. Find the value of the unknown interior angle x in the following figures:
Solution:
(i) ∠x + 50° = 115° (Exterior angle of a triangle)
∴ ∠x = 115°- 50° = 65°
(ii) ∠x + 70° = 110° (Exterior angle of a triangle)
∴ ∠x = 110° – 70° = 40°
(iii) ∠ x + 90° = 125° (Exterior angle of a right triangle)
∴ ∠x = 125° – 90° = 35°
(iv) ∠x + 60° = 120° (Exterior angle of a triangle)
∴ ∠x = 120° – 60° = 60°
(v)∠ X + 30° = 80° (Exterior angle of a triangle)
∴ ∠x = 80° – 30° = 50°
(vi) ∠ x + 35° = 75° (Exterior angle of a triangle)
∴ ∠ x = 75° – 35° = 40°
NCERT Solutions of class 7th maths Chapter 6 The Triangle and its Properties Exercise 6.3
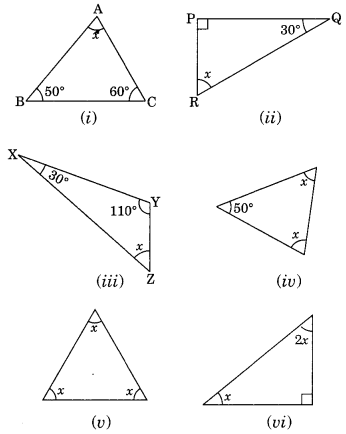
Q1. Find the value of the unknown x in the following diagrams:
Solution:
(i) By angle sum property of a triangle, we have
∠x + 50° + 60° = 180°
⇒ ∠x + 110° = 180°
∴ ∠x = 180° – 110° = 70°
(ii) By angle sum property of a triangle, we have
∠x + 90° + 30 = 180° [∆ is right angled triangle]
⇒ ∠x + 120° = 180°
∴ ∠x – 180° – 120° = 60°
(iii) By angle sum property of a triangle, we have
∠x + 30° + 110° – 180°
⇒ ∠x + 140° = 180°
∴ ∠x = 180° – 140° = 40°
(iv ) By angle sum property of a triangle, we have
∠x + ∠x + 50° = 180°
⇒ 2x + 50° = 180°
⇒ 2x = 180° – 50°
⇒ 2x = 130°
x = 130°/2
x = 65°
(v) By angle sum property of a triangle, we have
∠x + ∠x +∠x =180°
⇒ 3 ∠x = 180°
∠x = 180°/3
∠x = 60°
(vi) By angle sum property of a triangle, we have
x + 2 x + 90° = 180° (∆ is right angled triangle)
⇒ 3x + 90° = 180°
⇒ 3x = 180° – 90°
⇒ 3x = 90°
x = 90°/3
x = 30°
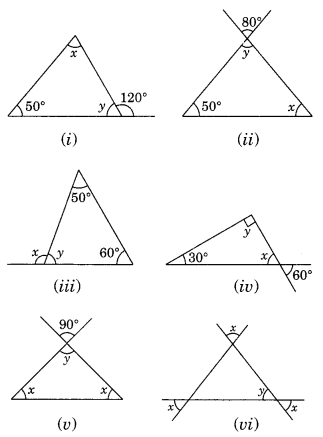
2. Find the values of the unknowns x and y in the following diagrams:
Solution:
(i) ∠x + 50° = 120° (Exterior angle of a triangle)
∴ ∠x = 120°- 50° = 70°
∠x + ∠y + 50° = 180° (Angle sum property of a triangle)
70° + ∠y + 50° = 180°
∠y + 120° = 180°
∠y = 180° – 120°
∴ ∠y = 60°
Thus ∠x = 70 and ∠y – 60°
(ii) ∠y = 80° (Vertically opposite angles are same)
∠x + ∠y + 50° = 180° (Angle sum property of a triangle)
⇒ ∠x + 80° + 50° = 180°
⇒ ∠x + 130° = 180°
∴ ∠x = 180° – 130° = 50°
Thus, ∠x = 50° and ∠y = 80°
(iii) ∠y + 50° + 60° = 180° (Angle sum property of a triangle)
∠y + 110° = 180°
∴ ∠y = 180°- 110° = 70°
∠x + ∠y = 180° (Linear pairs)
⇒ ∠x + 70° = 180°
∴ ∠x = 180° – 70° = 110°
Thus, ∠x = 110° and y = 70°
(iv) ∠x = 60° (Vertically opposite angles)
∠x + ∠y + 30° = 180° (Angle sum property of a triangle)
⇒ 60° + ∠y + 30° = 180°
⇒ ∠y + 90° = 180°
⇒ ∠y = 180° – 90° = 90°
Thus, ∠x = 60° and ∠y = 90°
(v) ∠y = 90° (Vertically opposite angles)
∠x + ∠x + ∠y = 180° (Angle sum property of a triangle)
⇒ 2 ∠x + 90° = 180°
⇒ 2∠x = 180° – 90°
⇒ 2∠x = 90°
∠x = 90°/2
∠x = 45°
(vi) From the rule of vertically opposite angles,
= x = y
Then,
We know that,
The sum of all the interior angles of a triangle is 180o.
Then,
= x + x + x = 180o
= 3x = 180o
= x = 180o/3
= x = 60o
NCERT Solutions of class 7th maths Chapter 6 The Triangle and its Properties Exercise 6.4
Q1. Is it possible to have a triangle with the following sides?
(i) 2 cm, 3 cm, 5 cm
Solution:-
Clearly, we have:
(2 + 3) = 5
5 = 5
Thus, the sum of any two of these numbers is not greater than the third.
Hence, it is not possible to draw a triangle whose sides are 2 cm, 3 cm and 5 cm.
(ii) 3 cm, 6 cm, 7 cm
Solution:-
Clearly, we have:
(3 + 6) = 9 > 7
(6 + 7) = 13 > 3
(7 + 3) = 10 > 6
Thus, the sum of any two of these numbers is greater than the third.
Hence, it is possible to draw a triangle whose sides are 3 cm, 6 cm and 7 cm.
(iii) 6 cm, 3 cm, 2 cm
Solution:-
Clearly, we have:
(3 + 2) = 5 < 6
Thus, the sum of any two of these numbers is less than the third.
Hence, it is not possible to draw a triangle whose sides are 6 cm, 3 cm and 2 cm.
2. Take any point O in the interior of a triangle PQR. Is
(i) OP + OQ > PQ?
(ii) OQ + OR > QR?
(iii) OR + OP > RP?
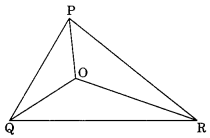
Solution:
(i) Yes, In ∆OPQ, we have
OP + OQ > PQ
[Sum of any two sides of a triangle is greater than the third side]
(ii) Yes, In ∆OQR, we have OQ + OR > QR
[Sum of any two sides of a triangle is greater than the third side]
(iii) Yes, In ∆OPR, we have OR + OP > RP
[Sum of any two sides of a triangle is greater than the third side]
3. AM is a median of a triangle ABC.
Is AB + BC + CA > 2 AM?
(Consider the sides of triangles ΔABM and ΔAMC.)
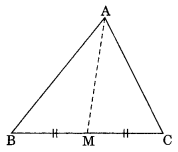
AB + BM > AM …(i)
[Sum of any two sides of a triangle is greater than the third side]
In ∆AMC, we have
AC + CM > AM …(ii)
[Sum of any two sides of a triangle is greater than the third side]
Adding eq (i) and (ii), we have
AB + AC + BM + CM > 2AM
AB + AC + BC + > 2AM
AB + BC + CA > 2AM Hence proved.
4. ABCD is a quadrilateral.
Is AB + BC + CD + DA > AC + BD?
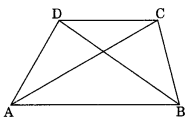
In ∆ABC, we have
AB + BC > AC …(i)
[Sum of any two sides is greater than the third side]
In ∆BDC, we have
BC + CD > BD …(ii)
[Sum of any two sides is greater than the third side]
In ∆ADC, we have
CD + DA > AC …(iii)
[Sum of any two sides is greater than the third side]
In ∆DAB, we have
DA + AB > BD …(iv)
[Sum of any two sides is greater than the third side]
Adding eq. (i), (ii), (iii) and (iv), we get
2AB + 2BC + 2CD + 2DA > 2AC + 2BD or AB + BC + CD + DA > AC + BD [Dividing both sides by 2]
Hence, proved.
5. ABCD is quadrilateral. Is AB + BC + CD + DA < 2 (AC + BD)
Solution:-
Yes, we have a quadrilateral ABCD.
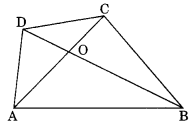
In ∆AOB, we have AB < AO + BO …(i)
[Any side of a triangle is less than the sum of other two sides]
In ∆BOC, we have
BC < BO + CO …(ii)
[Any side of a quadrilateral is less than the sum of other two sides]
In ∆COD, we have
CD < CO + DO …(iii)
[Any side of a triangle is less than the sum of other two sides]
In ∆AOD, we have
DA < DO + AO …(iv)
[Any side of a triangle is less than the sum of other two sides]
Adding eq. (i), (ii), (iii) and (iv), we have
AB + BC + CD + DA
∠2AO + ∠BO + ∠CO + ∠DO
∠2(AO + BO + CO + DO)
∠2 [(AO + CO) + (BO + DO)]
∠2(AC + BD)
Thus, AB + BC + CD + DA < 2(AC + BD)
Hence, proved.
6. The lengths of two sides of a triangle are 12 cm and 15 cm. Between what two measures should the length of the third side fall?
Solution:-
We know that,
The sum of the length of any two sides is always greater than the third side.
From the question, it is given that two sides of triangle are 12 cm and 15 cm.
So, the third side length should be less than the sum of other two sides,
12 + 15 = 27 cm.
Then, it is given that the third side is cannot not be less than the difference of the two sides, 15 – 12 = 3 cm
So, the length of the third side falls between 3 cm and 27 cm.
NCERT Solutions of class 7th maths Chapter 6 The Triangle and its Properties Exercise 6.5
Q1. PQR is a triangle, right-angled at P. If PQ = 10 cm and PR = 24 cm, find QR.
Solution:-
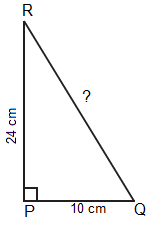
By the rule of Pythagoras Theorem,
Pythagoras theorem states that for any right angled triangle, the area of the square on the hypotenuse is equal to the sum of the areas of square on the legs.
In the above figure RQ is the hypotenuse,
QR2 = PQ2 + PR2
QR2 = 102 + 242
QR2 = 100 + 576
QR2 = 676
QR = √676
QR = 26 cm
Hence, the length of the hypotenuse QR = 26 cm.
2. ABC is a triangle, right-angled at C. If AB = 25 cm and AC = 7 cm, find BC.
Solution:-
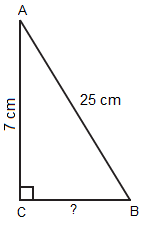
By the rule of Pythagoras Theorem,
Pythagoras theorem states that for any right angled triangle, the area of the square on the hypotenuse is equal to the sum of the areas of square on the legs.
In the above figure RQ is the hypotenuse,
AB2 = AC2 + BC2
252 = 72 + BC2
625 = 49 + BC2
By transposing 49 from RHS to LHS it becomes – 49
BC2 = 625 – 49
BC2 = 576
BC = √576
BC = 24 cm
Hence, the length of the BC = 24 cm.
3. A 15 m long ladder reached a window 12 m high from the ground on placing it against a wall at a distance a. Find the distance of the foot of the ladder from the wall.
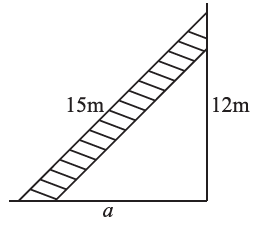
Solution:-
By the rule of Pythagoras Theorem,
Pythagoras theorem states that for any right angled triangle, the area of the square on the hypotenuse is equal to the sum of the areas of square on the legs.
In the above figure RQ is the hypotenuse,
152 = 122 + a2
225 = 144 + a2
By transposing 144 from RHS to LHS it becomes – 144
a2 = 225 – 144
a2 = 81
a = √81
a = 9 m
Hence, the length of a = 9 m.
4. Which of the following can be the sides of a right triangle?
(i) 2.5 cm, 6.5 cm, 6 cm.
(ii) 2 cm, 2 cm, 5 cm.
(iii) 1.5 cm, 2cm, 2.5 cm.
In the case of right-angled triangles, identify the right angles.
Solution:-
(i) Let a = 2.5 cm, b = 6.5 cm, c = 6 cm
Let us assume the largest value is the hypotenuse side i.e. b = 6.5 cm.
Then, by Pythagoras theorem,
b2 = a2 + c2
6.52 = 2.52 + 62
42.25 = 6.25 + 36
42.25 = 42.25
The sum of square of two side of triangle is equal to the square of third side,
∴The given triangle is right-angled triangle.
Right angle lies on the opposite of the greater side 6.5 cm.
(ii) Let a = 2 cm, b = 2 cm, c = 5 cm
Let us assume the largest value is the hypotenuse side i.e. c = 5 cm.
Then, by Pythagoras theorem,
c2 = a2 + b2
52 = 22 + 22
25 = 4 + 4
25 ≠ 8
The sum of square of two side of triangle is not equal to the square of third side,
∴The given triangle is not right-angled triangle.
(iii) Let a = 1.5 cm, b = 2 cm, c = 2.5 cm
Let us assume the largest value is the hypotenuse side i.e. b = 2.5 cm.
Then, by Pythagoras theorem,
b2 = a2 + c2
2.52 = 1.52 + 22
6.25 = 2.25 + 4
6.25 = 6.25
The sum of square of two side of triangle is equal to the square of third side,
∴The given triangle is right-angled triangle.
Right angle lies on the opposite of the greater side 2.5 cm.
5. A tree is broken at a height of 5 m from the ground and its top touches the ground at a distance of 12 m from the base of the tree. Find the original height of the tree.
Solution:-
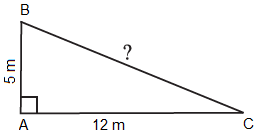
By observing the figure we came to conclude that right angle triangle is formed at A.
From the rule of Pythagoras theorem,
BC2 = AB2 + AC2
BC2 = 52 + 122
BC2 = 25 + 144
BC2 = 169
BC = √169
BC = 13 m
Then, the original height of the tree = AB + BC
= 5 + 13
= 18 m
6. Angles Q and R of a ΔPQR are 25o and 65o.
Write which of the following is true:
(i) PQ2 + QR2 = RP2
(ii) PQ2 + RP2 = QR2
(iii) RP2 + QR2 = PQ2
Solution:-
Given that ∠B = 35o, ∠C = 55o
Then, ∠A =?
We know that sum of the three interior angles of triangle is equal to 180o.
= ∠PQR + ∠QRP + ∠RPQ = 180o
= 25o + 65o + ∠RPQ = 180o
= 90o + ∠RPQ = 180o
= ∠RPQ = 180 – 90
= ∠RPQ = 90o
Also, we know that side opposite to the right angle is the hypotenuse.
∴ QR2 = PQ2 + PR2
Hence, (ii) is true
7. Find the perimeter of the rectangle whose length is 40 cm and a diagonal is 41 cm.
Solution:-
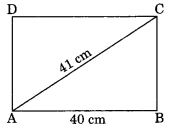
Let ABCD be the rectangular plot.
Then, AB = 40 cm and AC = 41 cm
BC =?
According to Pythagoras theorem,
From right angle triangle ABC, we have:
= AC2 = AB2 + BC2
= 412 = 402 + BC2
= BC2 = 412 – 402
= BC2 = 1681 – 1600
= BC2 = 81
= BC = √81
= BC = 9 cm
Hence, the perimeter of the rectangle plot = 2 (length + breadth)
Where, length = 40 cm, breadth = 9 cm
Then,
= 2(40 + 9)
= 2 × 49
= 98 cm
8. The diagonals of a rhombus measure 16 cm and 30 cm. Find its perimeter.
Solution:-
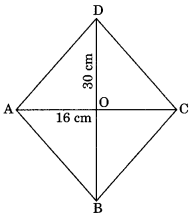
Solution:
Let ABCD be a rhombus whose diagonals intersect each other at O such that AC = 16 cm and BD = 30 cm
Since, the diagonals of a rhombus bisect each other at 90°.
∴ OA = OC = 8 cm and OB = OD = 15 cm
In right ∆OAB,
AB2 = OA2 + OB2 (By Pythagoras property)
= (8)2+ (15)2 = 64 + 225
= 289
∴ AB =[latex]sqrt{289}[/latex]= 17 cm
Since AB = BC = CD = DA (Property of rhombus)
∴ Required perimeter of rhombus
= 4 × side = 4 × 17 = 68 cm
